Introduction
A geometry connector is a small device that, used along with other similar ones, allows to connect straws and to create geometrical forms.
Available straws which exist on the market come in a great variety of diameters – from 3.5mm to 12mm or even 14mm. 3D printed connectors present the benefit of being customizable; they can be printed so that they fit any straw diameter.Étape 1 - Description of the object that we have prototyped
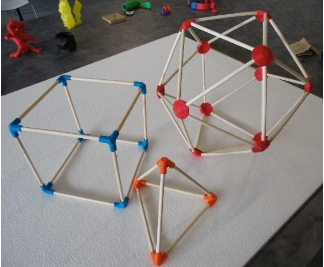
The objects we have prototyped are several different 3D printed geometrical straw connectors, allowing the construction of different geometrical shapes. They are easily done and cost-effective: it takes about 15 minutes of printing to achieve one item. It is possible to modify the design in order to adapt it to different straw diameters, and to different numbers of connected straws. These 3D printed straw connectors can be printed by designers, amateurs, mathematics and geometry teachers, or even pupils/ students, under appropriate supervision.
Étape 2 - For further discussion and work
The 3D printed geometry connectors that we have prototyped can serve to introduce children and students to 3D printing and to the study of geometrical shapes, being extremely useful in the context of formal and non-formal learning and education. They can be used in geometry and mathematics classes in order to enhance the sense of space, spatial planning, spatial thinking and geometrical thinking in students. They can accompany and/ or replace (as considered suitable by teachers) the classical drawings on paper when solving of geometry problems, facilitating visualization and creative thinking.
Notes et références
A romanian version of this publication is available here.
This tutorial was produced as part of the FabEdu project, co-financed by the Erasmus + Programme of the European Union.
Project number: 2017-1-FR02-KA205-012767
The content of this publication does not reflect the official opinion of the European Union. Responsibility for the information and views expressed therein lies entirely with the author(s).
Published

 Français
Français English
English Deutsch
Deutsch Español
Español Italiano
Italiano Português
Português